Subsections
Lecture Notes
The information in this section (and in Lab Manual Appendix C) is
supplementary to the lecture part of the course, and contains material
from a few of the lectures that is not contained in the
textbook. It is included here to free students from having to
meticulously copy formulae, and should be brought to lectures when it
is being discussed in class. Half of the page is left blank, so that
students can add their own notes or diagrams from reading and/or
lecture material.
Energy and its Dimensions and Units
Energy is ``the ability to do work''. We can express energy in units
of work, and there are several systems of units for doing this (see
Lab Manual, Appendix D). We use the S.I. system in this course. There
are 4 base dimensions from which all other quantities can be derived:
length (L), mass (M), time (T) and temperature ( ). The
important derived quantities are (dimensions are in parentheses and
the corresponding S.I. units in square brackets):
). The
important derived quantities are (dimensions are in parentheses and
the corresponding S.I. units in square brackets):
- Force is the ``push'' required to accelerate unit mass (M)
[kg] at a unit of distance per unit time per unit time (M L
T-2) [kg m
s-2 = 1N (Newton)].
- Work or Energy is a unit of force (M L
T-2)
[kg m
s-2 = 1N (Newton)] displaced through a unit of distance:
(M
L2
T-2 = Q) [ kg
m2
s-2 = N m = 1 J (Joule)].
- Power or Heat flux is the energy flow per time or
the rate of energy flow (rate of work) (M
L2
T-3 = Q
T-1) [ J
s-1 = 1 W (Watt)].
- Heat Flux Density is rate of energy gained or lost unit
surface area (M
T-3 = Q
L-2
T-1) [W
m-2].
Energy can be found in many forms, the 5 of greatest
significance in climatic applications are:
- RADIANT ENERGY: energy associated with electromagnetic waves,
requires no medium (i.e. can travel through space)
- KINETIC ENERGY: energy due to motion:
KE =
 so, (KE) =
(M
L2
T-2 = Q)
so, (KE) =
(M
L2
T-2 = Q)
- GEOPOTENTIAL ENERGY: energy derived from gravity:
GPE = mgh
m = mass (M), g = gravitational acceleration (L
T-2),
h = height (L)
 (GPE) = (M L
T-2 L) = M
L2
T-2 = Q)
(GPE) = (M L
T-2 L) = M
L2
T-2 = Q)
- INTERNAL ENERGY: sensible heat (heat that can be felt) of a body
due to random motion of molecules:
IE = mcT
m = mass (M), c = specific heat (Q
M-1
 ) T =
temperature (
) T =
temperature ( )
)
 (IE) = (M Q
M-1
(IE) = (M Q
M-1
 = Q)
= Q)
- LATENT HEAT: energy involved in phase changes of a substance,
especially water:
LE = mL
m = mass (M), L = latent heat (Q
M-1)
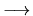 (LE)
= (M Q
M-1 = Q)
(LE)
= (M Q
M-1 = Q)
Energy can be neither created nor destroyed, but it can be converted
from one form to another (i.e. between forms 1-5 above).
Example: Solar radiation (form 1), heats surface (4), warms air which
rises (2 and 3) and evaporates water (5).
- Radiation -- heat transfer due to rapid oscillations of
electromagnetic fields. May also be considered as waves.
- Conduction -- heat transfer by internal molecular
activity within a substance with no net external motion. Requires
contact between molecules in a substance. Solids, especially metals,
are good conductors of heat while liquids and gases are poor due to
lower molecular density. In atmosphere conduction is negligible except
within the first few millimeters from a surface.
- Convection -- heat (and mass) transfer within a fluid by
mass motion resulting in transport and mixing. Convection is very
important in the atmosphere. Two types of convective motion exist:
- Free - buoyancy due to thermal differences (war air is less
dense, so will rise; cold air is more dense and will sink).
- Forced - due to physical overturning via shear (air flowing
over a rough surface induces vertical motion).
Radiant energy incident on a body may be reflected,
transmitted or absorbed, so that:
where
r = reflectivity (the fraction of incident radiation
that is reflected),
t
= reflectivity (the fraction of incident radiation
that is reflected),
t = transmissivity (the fraction
transmitted),
a
= transmissivity (the fraction
transmitted),
a = absorptivity (the fraction absorbed), of
the body for radiation of wave-length
= absorptivity (the fraction absorbed), of
the body for radiation of wave-length  . This can also be
applied to bands of many wavelengths. For the band of solar radiation
wavelengths,
r
. This can also be
applied to bands of many wavelengths. For the band of solar radiation
wavelengths,
r is called the albedo (
is called the albedo ( ).
).
Relates the way in which the ``emissive power'' (total energy emitted
by a body) of a black body (a perfect emitter) is dependent upon its
temperature at all wavelengths. This is the law from which
Wien's Law and the Stephan-Boltzmann Law are derived.
States that a rise in temperature of a body increases its
emissive power and also increases the proportion of shorter wave
lengths which it emits:
=
where
 = wavelength of maximum emission (m)
T0 = surface temperature (K)
= wavelength of maximum emission (m)
T0 = surface temperature (K)
States that the total energy emitted by a black body, integrated over
all wavelengths, is proportional to the fourth power of its absolute
temperature (Temperature in Kelvins):
I =
 T04
T04
where I = energy emitted by the black body,  =
Stefan-Boltzmann constant
= 5.67 x 10-8W
m-2
K-4
=
Stefan-Boltzmann constant
= 5.67 x 10-8W
m-2
K-4
For non-blackbodies inclusion of the surface emissivity
(
 ) allows calculation of the emission:
) allows calculation of the emission:
E =
 T04
T04
Assuming no transmission through a body, it follows that for a given
wavelength and temperature the absorptivity of a body equals its
emissivity:
a
=
The solar constant (I0) is the amount of solar radiation
received from outside the Atmosphere in one unit time on one unit
surface area placed perpendicular to the solar beam (as in plane CD in the
diagram below) at the Earth's mean distance from the Sun. Present
estimates suggest a value of 1367 W
m-2 which therefore
represents the upper limit for solar radiation receipt in the
Earth-Atmosphere system. Only locations where the Sun can be directly
overhead can receive this value (e.g. in the Tropics). The
extra-terrestrial solar radiation (I) received at all other
latitudes is less than I0 and is given by the cosine law of
illumination. For example I on the plane AB (representing the top
of the Atmosphere) in the following figure is given:
I = I0cosZ
Figure 1:
Radiation incident on a surface.
|
|
where I0 = solar radiation received on plane CD perpendicular to beam
(i.e. conforms to definition of solar constant), Z = angle between
the beam and a perpendicular to the surface (zenith angle);
The zenith angle depends on latitude, season and time of day and gives
rise to considerable variation in the amount of energy received over
the exterior of the planet.
Further variations in solar radiation receipt at the surface are due
to differing path lengths of radiation through the atmosphere due to
Earth-Sun geometry and the effectiveness of atmospheric attenuation.
- Absorption: the atmosphere is a relatively poor and
selective absorber of shortwave. The principal agents are O3,
cloud droplets, particles and water vapour.
- Scattering: small gaseous molecules scatter or diffuse
shortwave radiation. The shortest wavelengths are preferentially
scattered.
- Reflection: like a mirror, solar radiation is reflected
from larger particles and dominantly by clouds. The reflectivity,
or albedo (
 , ranges between 0 and 1 with a maximum value of
1 for a perfect reflector like a mirror) of cloud tops is between
0.4 and 0.8 with a mean of 0.55.
, ranges between 0 and 1 with a maximum value of
1 for a perfect reflector like a mirror) of cloud tops is between
0.4 and 0.8 with a mean of 0.55.
Solar radiation reaching the surface (
K  ) has 2
components: direct-beam (S) as a parallel stream from the solar
disc, and diffuse (D) from all points of the sky hemisphere (having
been scattered and reflected during passage through the Atmosphere)
so that:
) has 2
components: direct-beam (S) as a parallel stream from the solar
disc, and diffuse (D) from all points of the sky hemisphere (having
been scattered and reflected during passage through the Atmosphere)
so that:
K 
=
S +
D
For opaque surfaces (transmissivity
t = 0) shortwave radiation is
either reflected or absorbed. Reflection (
K
= 0) shortwave radiation is
either reflected or absorbed. Reflection (
K  ) depends on
the amount of incident radiation (
K
) depends on
the amount of incident radiation (
K  ) and the surface
albedo (
) and the surface
albedo ( ):
):
The absorbed (or net) shortwave radiation at the surface (K*) is
therefore:
All bodies with temperature above absolute zero radiate energy
consistent with their surface temperature and surface emissivity as
given by Stefan-Boltzmann's Law:
E =
 T04
T04
At temperatures typical in the Earth-Atmosphere system the wave-length
of emission of a body corresponds to infra-red or longwave radiation.
The Atmosphere is a relatively good (but still selective) absorber of
this longwave radiation. Important absorbers are: water vapour (5-8
 m and at > 15
m and at > 15 m); CO2 (13-17
m); CO2 (13-17  m and 4.3
m and 4.3  m);
O3 (9.4-9.8
m);
O3 (9.4-9.8  m and 15
m and 15  m); and cloud droplets / particles
at almost all longwave wavelengths.
m); and cloud droplets / particles
at almost all longwave wavelengths.
There is a significant gap between 8 and 13  m - called the
``atmospheric window''. Overall the atmosphere is a relatively good
absorber of longwave radiation allowing comfortable living conditions
to exist on Earth, hence the so-called Greenhouse (or better
Atmosphere) Effect. The Atmosphere largely allows
shortwave in but effectively traps a lot of longwave emitted from the
surface. This results in a warming of the atmosphere which increases
the
L
m - called the
``atmospheric window''. Overall the atmosphere is a relatively good
absorber of longwave radiation allowing comfortable living conditions
to exist on Earth, hence the so-called Greenhouse (or better
Atmosphere) Effect. The Atmosphere largely allows
shortwave in but effectively traps a lot of longwave emitted from the
surface. This results in a warming of the atmosphere which increases
the
L  (back radiation) from the atmosphere to the
Earth's surface. The back radiation effectively warms the average
surface temperature by 33 K over what it would be if the atmosphere
did not exist.
(back radiation) from the atmosphere to the
Earth's surface. The back radiation effectively warms the average
surface temperature by 33 K over what it would be if the atmosphere
did not exist.
Kirchhoff's law tells us that a good absorber is a good radiator at
the same wavelength. The Atmosphere radiates longwave, some to space
and some to the ground (
L  ). The surface also radiates
longwave to the atmosphere (
L
). The surface also radiates
longwave to the atmosphere (
L  ).
).
The surface longwave radiation budget therefore consists of two
components:
L* =
L 
-
L 
if the surface is a black body ( = 1):
= 1):
L* =
L 
-
 T04
T04
but if the surface is a `grey body' with
 < 1.0 we need to
allow for less emission but some reflection (reflection =
(1 -
< 1.0 we need to
allow for less emission but some reflection (reflection =
(1 -  )L
)L  ):
):
L* =
L 
- (
 T04
T04 + (1 -
)
L 
)
Usually
L  < L
< L  therefore L* is
negative. With clear skies L* is typically about -100 W
m-2 for surfaces with an unobstructed view of the sky. The
addition of cloud and/or horizon obstructions (e.g. trees, leaves,
buildings, topography) substantially lessens or even reverses the
radiation losses by increasing
L
therefore L* is
negative. With clear skies L* is typically about -100 W
m-2 for surfaces with an unobstructed view of the sky. The
addition of cloud and/or horizon obstructions (e.g. trees, leaves,
buildings, topography) substantially lessens or even reverses the
radiation losses by increasing
L  .
.
The net all-wave radiative budget of a surface (i.e. whether it is
gaining or losing energy over all wavelengths) is the net result of
the short- and longwave budgets, so that during the day:
| Q* |
= |
K* + L* |
|
| |
= |
K  - K - K  + L + L  - L - L  |
|
At night
Q* = L* as K* = 0. Normally by day the radiative budget of
a surface is in surplus (Q* is positive) and by night in deficit
(Q* is negative).
Since a surface is a ``massless plane'' (so thin that it has no mass)
it can have no heat content and therefore the Law of Conservation of
Energy requires that the radiative energy imbalances be dissipated.
This is accomplished via convection or conductive exchanges towards or
away from the surface:
Q* = QH + QE + QG
where
QH, QE are the convective transfers of sensible and
latent heat to or from the atmosphere respectively and QG is the
conductive transfer of sensible heat to or from the ground. Each of
these fluxes may be an energy gain (when directed towards) or a loss
(directed away) for the surface. The sign convention for
QH, QE, QG is that positive values represent energy flows away from
the surface, and negative values represent energy flows toward
the surface. Thus a surplus of radiant energy at the surface (positive
Q*) results in the flow of sensible or latent heat away from the
surface (positive QH, QE and/or QG). In moist environments the
daytime radiative energy surplus is primarily dissipated as latent
heat through the evaporation of water from the surface (QE).
A continuous transfer of water occurs through transport and phase
changes between subsystems of the Earth-Atmosphere system. Water is
evapotranspired (evaporated and transpired through plant respiration)
into the atmosphere in response to the local energy balance. Uplift
leads to cooling, condensation and precipitation (rain, snow, etc.)
thus returning the water to the surface again. There are also
transports of water within the atmosphere by advection, across land
by river run-off, and through the ground as ground water.
| Surface plane |
p = E + f +  r r |
| Soil column |
p = E +  r + r +  S S |
where, p = precipitation, E = evapotranspiration, f =
infiltration,  r - net runoff and
r - net runoff and  S net soil moisture
storage. Usually p is the sole input, the other terms are outputs
or storage terms, but it is possible for E (as dewfall),
S net soil moisture
storage. Usually p is the sole input, the other terms are outputs
or storage terms, but it is possible for E (as dewfall),  r,
or irrigation to be inputs.
r,
or irrigation to be inputs.
The Energy and Water Balances are linked by the energy required to
change the phase of water:
QE = LVE
where, LV = latent heat of vaporization.
Since p is not a continuous input but occurs as an on/off process
Water Budgets normally refer to periods of few days or longer.
Our understanding of convective transfer is greatly aided by knowledge
of 3 simple thermodynamic laws: Ideal Gas Law; Hydrostatic Law;
1st Law of Thermodynamics
- Ideal Gas Law (Equation of State for the Atmosphere)
Gases consist of minute molecules in a state of irregular
motion. The pressure (P) of a gas results from the impacts of these
molecules and for one unit volume of gas, depends on:
- number of molecules
- mass of molecules
- speed of molecules
a) and b) define the density ( ) of the gas (ie.
no. x
) of the gas (ie.
no. x 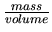 ), and c) depends upon the gases temperature (T).
These are related by:
), and c) depends upon the gases temperature (T).
These are related by:
P 
 T
T
or
P =
R T
T
where R is the Specific Gas Constant (
R =  where
R* is the universal gas constant = 8.314 kg
m2
s-2
mole-1
K-1; and Mair is the molecular weight of
``air'' = .028 kg
mole-1
(This is the same as the more familiar form: PV = n
R*T, except
that n, the number of moles of gas is replaced by
where
R* is the universal gas constant = 8.314 kg
m2
s-2
mole-1
K-1; and Mair is the molecular weight of
``air'' = .028 kg
mole-1
(This is the same as the more familiar form: PV = n
R*T, except
that n, the number of moles of gas is replaced by
 where m is the mass of gas under consideration.)
where m is the mass of gas under consideration.)
- Hydrostatic Law
Consider an air column divided
into thin horizontal slices of thickness  z with cross
sectional area a. Then the volume of a slice is
a
z with cross
sectional area a. Then the volume of a slice is
a z. If
z. If  is air density, the mass of the slice is
is air density, the mass of the slice is
 a
a z. If the acceleration due to gravity is g, the force on the slice (F=ma) is
g
z. If the acceleration due to gravity is g, the force on the slice (F=ma) is
g a
a z and the pressure (pressure is force per unit area) at A due to the slice
is
z and the pressure (pressure is force per unit area) at A due to the slice
is
 = g
= g
 z. Thus the
pressure difference
z. Thus the
pressure difference  P decreases with height:
P decreases with height:
where the sign indicates that P
decreases as z increases. This is the Hydrostatic Equation, and is
also a statement of hydrostatic balance: the vertical decrease of
pressure tending to cause uplift is balanced by the downward weight
of the air. Hence there is no vertical acceleration and the
atmosphere does not float away (luckily!).
- 1st Law of Thermodynamics
This is a Law of Conservation of Energy, and a statement of the physical
changes resulting when heat is added to, or taken away from a gas. For
solids, recall that there is a direct relationship between the heat
added ( Q) and the corresponding temperature change (
Q) and the corresponding temperature change ( T):
T):
With a gas we have to consider whether the gas is capable of
expansion. If not, (ie. V is constant) addition of heat will result
in a greater value of  T than if it is able to expand because
in the latter case some of the energy is used to do the work of
expansion. So for gases we have two specific heats: one for constant
volume (CV), and the other for constant pressure (CP), and
the 1st Law of Thermodynamics is:
T than if it is able to expand because
in the latter case some of the energy is used to do the work of
expansion. So for gases we have two specific heats: one for constant
volume (CV), and the other for constant pressure (CP), and
the 1st Law of Thermodynamics is:
 Q Q |
= |
CV T T |
+ |
P/(  ) ) |
 Q Q |
= |
CP T T |
- |
( P)/ P)/ |
| change |
= |
change in |
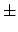 |
work due to |
| in heat |
|
internal energy |
|
expansion |
The second form, involving CP is most useful, because the changes
 T, and
T, and  P are easy to measure, whereas
P are easy to measure, whereas

 is not. The value of CP for air is 1010 J
kg-1
K-1.
CP, CV, and R (the gas constant for air) are related by:
is not. The value of CP for air is 1010 J
kg-1
K-1.
CP, CV, and R (the gas constant for air) are related by:
| R |
= |
CP |
- |
CV |
| 287 |
= |
1010 |
- |
723 [J
kg-1
K-1] |
- What primary laws of physics does atmospheric motion obey?
- What are meant by real and apparent forces?
- What real forces are important in the atmosphere? What is
their affect?
- What apparent forces are important in the atmosphere?
What is their affect?
- How does wind (horizontal motion) result from a balance of the
forces acting on an air parcel?
- Geostrophic, Gradient, and Cyclostrophic winds are
approximations to the full equation of motion. When are each of
these valid, and what are the assumptions upon which these models
are based?
Motion in the atmosphere behaves according to Newton's First Law:
In the absence of forces, a body in motion will remain in motion.
but it is Newton's second law that provides the basis for the
equations of motion:
=
m
which can be re-expressed as:
where
 = velocity,
= velocity,
 = acceleration,
= acceleration,
 = force, and m = mass. So, what are the important
forces in the atmosphere?
= force, and m = mass. So, what are the important
forces in the atmosphere?
Real forces:
Apparent forces are due to the earth's rotation. The earth is
a non-inertial frame of reference -- the frame of reference itself is
accelerating because of the rotation. Because of this, we must add
these apparent forces to the equation of motion on a sphere:
- Coriolis Force (
 ). The Coriolis force varies
with latitude, is proportional to and acts to the right of the
velocity (in the northern hemisphere). It is expressed as fV, where
f is called the Coriolis parameter and is equal to 2 times the
rotation rate of the earth times the sine of the latitude. [NOTE: The
textbook uses the symbol FC to represent
). The Coriolis force varies
with latitude, is proportional to and acts to the right of the
velocity (in the northern hemisphere). It is expressed as fV, where
f is called the Coriolis parameter and is equal to 2 times the
rotation rate of the earth times the sine of the latitude. [NOTE: The
textbook uses the symbol FC to represent
 .]
.]
- Centrifugal Force. The centrifugal force is included in gravity.
The force balance in the atmosphere relates the acceleration of an air
parcel to the sum of forces acting on it:
If we use a natural coordinate system, where s is the
coordinate along the direction of motion, n is the coordinate to the
left and perpendicular to the direction of motion,
 points in the direction of s, and
points in the direction of s, and
 points in the
direction of n.
points in the
direction of n.
Figure:
Natural coordinate system.  represents the wind
vector.
represents the wind
vector.  is the unit vector pointing in the direction of
motion.
is the unit vector pointing in the direction of
motion.  is the unit vector pointing perpendicular to the
direction of motion.
is the unit vector pointing perpendicular to the
direction of motion.
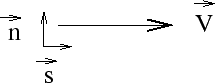 |
=
V
V =
and
where
=
V
is a centrifugal force for curved flow, and is equal to
where R is the radius of curvature and is
> 0 for curvature to the left and < 0 for curvature to the right.
So we have, in natural coordinates:
How do the forces fit into the natural coordinate system?
The Pressure Gradient Force
 can have components along and across the direction of
motion:
can have components along and across the direction of
motion:
The Friction Force
 always acts in a direction opposite
to the velocity:
always acts in a direction opposite
to the velocity:
= -
Ff
The Coriolis Force
 always acts to the right of the
motion (in the northern hemisphere):
always acts to the right of the
motion (in the northern hemisphere):
= -
fV
So, if we add all of these forces up, and write them as two equations,
one with force components in the direction of motion
 , and one with
force components perpendicular to the direction of motion
, and one with
force components perpendicular to the direction of motion
 we
get,
the force balance:
we
get,
the force balance:
which in the
 direction (along the flow) gives:
and in the
direction (along the flow) gives:
and in the
 direction (across the flow):
direction (across the flow):
Imagine an initial state with a horizontal pressure gradient and no
motion. Equation 2 implies that
 = 0 therefore, the initial flow will be down the pressure
gradient (across the isobars). As time proceeds, V becomes non-zero,
and the wind accelerates until a three way balance exists between
= 0 therefore, the initial flow will be down the pressure
gradient (across the isobars). As time proceeds, V becomes non-zero,
and the wind accelerates until a three way balance exists between
 ,
, , and
, and
 .
.
Assume that the flow is straight (ie.
R 
 ),
steady (ie.
),
steady (ie.
 = 0), and frictionless (Ff = 0).
These conditions will generally be met to within a few percent above a
couple of kilometers, and in regions where the isobars are straight.
Equation 1 implies that
= 0), and frictionless (Ff = 0).
These conditions will generally be met to within a few percent above a
couple of kilometers, and in regions where the isobars are straight.
Equation 1 implies that
 = 0 which means that the flow
is parallel to the isobars. Equation 2 implies that the Pressure gradient
force is balanced by the Coriolis force:
= 0 which means that the flow
is parallel to the isobars. Equation 2 implies that the Pressure gradient
force is balanced by the Coriolis force:
fV = -
which is the Geostrophic Wind relationship.
An alternate expression can be found for the geostrophic wind by using
the height gradient form of the pressure gradient force:
fV =
g
The gradient wind approximation relaxes the requirement for straight flow.
Again, equation 1 implies flow parallel to the isobars. For anti-clockwise
curvature (R > 0) (cyclonic) equation 2 is:
and for clockwise curvature (R < 0) (anticyclonic) equation 2
becomes:
So, for the same pressure gradient, anticyclonically curved flow will
have stronger winds than straight flow, which will have stronger winds
that cyclonically curved flow. However in practice, pressure gradients
are weaker around anticyclones.
Imagine, a curved circulation which exists on small time and length
scales, ignoring friction. In this case, we can ignore the Coriolis
force. The pressure gradient force is balanced by the centrifugal
force. The flow can be either cyclonic or anticyclonic.
This equation describes the circulation in a tornado.
Copyright © 2020 by Peter L. Jackson
![]() = reflectivity (the fraction of incident radiation
that is reflected),
t
= reflectivity (the fraction of incident radiation
that is reflected),
t![]() = transmissivity (the fraction
transmitted),
a
= transmissivity (the fraction
transmitted),
a![]() = absorptivity (the fraction absorbed), of
the body for radiation of wave-length
= absorptivity (the fraction absorbed), of
the body for radiation of wave-length ![]() . This can also be
applied to bands of many wavelengths. For the band of solar radiation
wavelengths,
r
. This can also be
applied to bands of many wavelengths. For the band of solar radiation
wavelengths,
r![]() is called the albedo (
is called the albedo (![]() ).
).
![]() ) allows calculation of the emission:
) allows calculation of the emission:
![]() ) has 2
components: direct-beam (S) as a parallel stream from the solar
disc, and diffuse (D) from all points of the sky hemisphere (having
been scattered and reflected during passage through the Atmosphere)
so that:
) has 2
components: direct-beam (S) as a parallel stream from the solar
disc, and diffuse (D) from all points of the sky hemisphere (having
been scattered and reflected during passage through the Atmosphere)
so that:
![]() = 0) shortwave radiation is
either reflected or absorbed. Reflection (
K
= 0) shortwave radiation is
either reflected or absorbed. Reflection (
K ![]() ) depends on
the amount of incident radiation (
K
) depends on
the amount of incident radiation (
K ![]() ) and the surface
albedo (
) and the surface
albedo (![]() ):
):
![]() m and at > 15
m and at > 15![]() m); CO2 (13-17
m); CO2 (13-17 ![]() m and 4.3
m and 4.3 ![]() m);
O3 (9.4-9.8
m);
O3 (9.4-9.8 ![]() m and 15
m and 15 ![]() m); and cloud droplets / particles
at almost all longwave wavelengths.
m); and cloud droplets / particles
at almost all longwave wavelengths.
![]() m - called the
``atmospheric window''. Overall the atmosphere is a relatively good
absorber of longwave radiation allowing comfortable living conditions
to exist on Earth, hence the so-called Greenhouse (or better
Atmosphere) Effect. The Atmosphere largely allows
shortwave in but effectively traps a lot of longwave emitted from the
surface. This results in a warming of the atmosphere which increases
the
L
m - called the
``atmospheric window''. Overall the atmosphere is a relatively good
absorber of longwave radiation allowing comfortable living conditions
to exist on Earth, hence the so-called Greenhouse (or better
Atmosphere) Effect. The Atmosphere largely allows
shortwave in but effectively traps a lot of longwave emitted from the
surface. This results in a warming of the atmosphere which increases
the
L ![]() (back radiation) from the atmosphere to the
Earth's surface. The back radiation effectively warms the average
surface temperature by 33 K over what it would be if the atmosphere
did not exist.
(back radiation) from the atmosphere to the
Earth's surface. The back radiation effectively warms the average
surface temperature by 33 K over what it would be if the atmosphere
did not exist.
![]() ). The surface also radiates
longwave to the atmosphere (
L
). The surface also radiates
longwave to the atmosphere (
L ![]() ).
).
![]() < L
< L ![]() therefore L* is
negative. With clear skies L* is typically about -100 W
m-2 for surfaces with an unobstructed view of the sky. The
addition of cloud and/or horizon obstructions (e.g. trees, leaves,
buildings, topography) substantially lessens or even reverses the
radiation losses by increasing
L
therefore L* is
negative. With clear skies L* is typically about -100 W
m-2 for surfaces with an unobstructed view of the sky. The
addition of cloud and/or horizon obstructions (e.g. trees, leaves,
buildings, topography) substantially lessens or even reverses the
radiation losses by increasing
L ![]() .
.
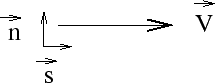
![]() can have components along and across the direction of
motion:
can have components along and across the direction of
motion:
![]() = 0 therefore, the initial flow will be down the pressure
gradient (across the isobars). As time proceeds, V becomes non-zero,
and the wind accelerates until a three way balance exists between
= 0 therefore, the initial flow will be down the pressure
gradient (across the isobars). As time proceeds, V becomes non-zero,
and the wind accelerates until a three way balance exists between
![]() ,
,![]() , and
, and
![]() .
.
![]()
![]() ),
steady (ie.
),
steady (ie.
![]() = 0), and frictionless (Ff = 0).
These conditions will generally be met to within a few percent above a
couple of kilometers, and in regions where the isobars are straight.
Equation 1 implies that
= 0), and frictionless (Ff = 0).
These conditions will generally be met to within a few percent above a
couple of kilometers, and in regions where the isobars are straight.
Equation 1 implies that
![]() = 0 which means that the flow
is parallel to the isobars. Equation 2 implies that the Pressure gradient
force is balanced by the Coriolis force:
= 0 which means that the flow
is parallel to the isobars. Equation 2 implies that the Pressure gradient
force is balanced by the Coriolis force: